Chord Naming – Name Every Chord!
The practical way to learn how to name chords is to begin with a set of base chords and build onto them, naming each variation until you build a vocabulary. Eventually, you can understand where chord names come from and use your knowledge to name any chord. You can also compare chords you see with those from the known set of base chords and variations.

Base Chords
In order to arrive at a good set of base chords, we will start with the dominant triad. In the key of C, this is G (or “G Major”, the major triad with root G, third B, and fifth D). As we build the set of base chords, you will see why we used the dominant. In the key of C, we won’t have to add any accidentals or think about sharps or flats in the base chords.
Special Convention for 7th Chords
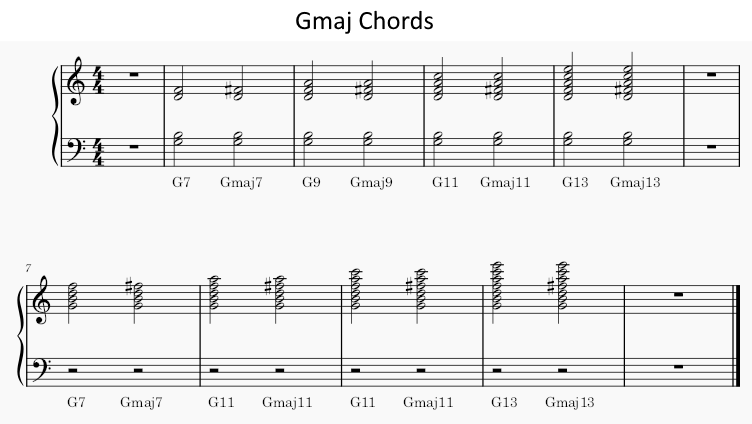
The second chord in our series of base chords in the G7. This is the G chord with an added flat seventh. In the context of chord naming, the 7 means ♭7. In the context of intervals in composition, “7th” would mean major seventh but in chord naming, this means ♭7 or a minor 7th interval from the root. This may not make a lot of sense but is just the convention that you have to remember.
The next chords in our series are build on the 7th and are the G9, G11, and G13, each one built on the previous. So, for example, the G13 contains the triad, the ♭7, 9th, 11th, and 13th.
You have probably realised already that the 9th is equivalent to a 2nd interval, the 11th is equivalent to a perfect 4th, and the 13th is equivalent to a 6th.
Maj7 Chords
To notate a chord with a major 7th, the term, “maj” is used. For example, Gmaj7. The term, “maj” applies to the 7th. For example, “Gmaj9” means a 9th chords (like in our base collection) with a major 7th instead of a minor 7th. Sometimes, a triangle, “Δ “ is used in place of “maj”
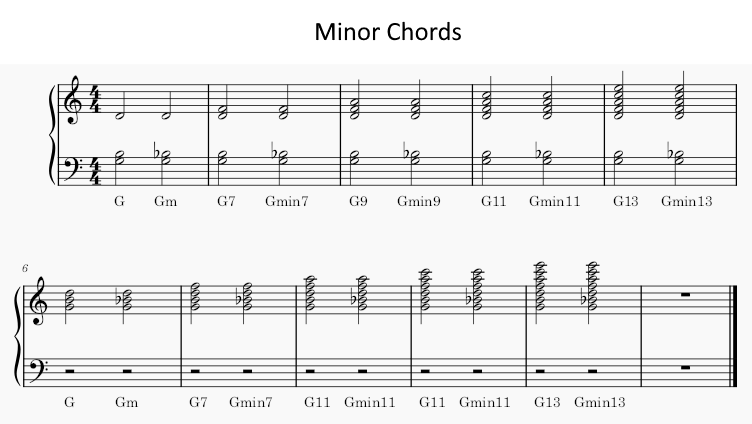
Minor Chords
The term “min” or “m” always refers to the third. For example, “Gm11” is an 11th chord with a minor third instead of a major third. Major and minor can be combined, since they refer to different notes. For example, “GminMaj11” is a G11 chord with the third lowered to a minor and the flat 7th raised to make a major 7th.
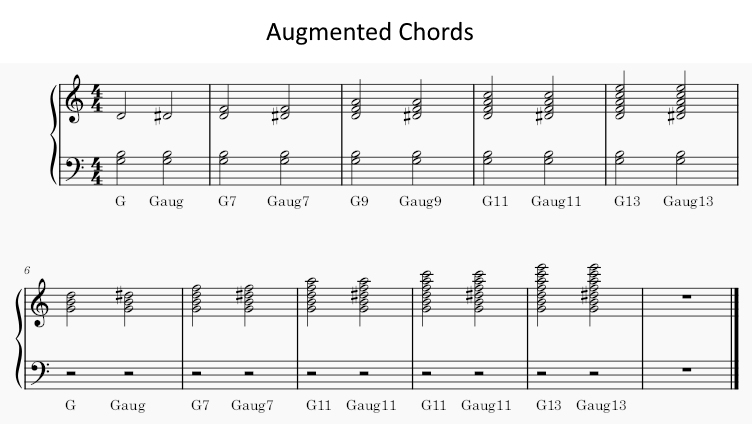
Augmented Chords
Augmented chords are denoted by “aug” or “+” or (#5). This means the fifth of the chord has been raised to a #5th.
For example, G7(#5) = Gaug7 = G+7
Flat Five Chords
Written as, for example, G7(♭5) or Gm11(♭5).
There is a special example of the flat five, which is the minor 7th flat 5, “Gm7(♭5)”. Due to its popularity, it has the name, “half diminished” and symbol, ø. For example, Gmø
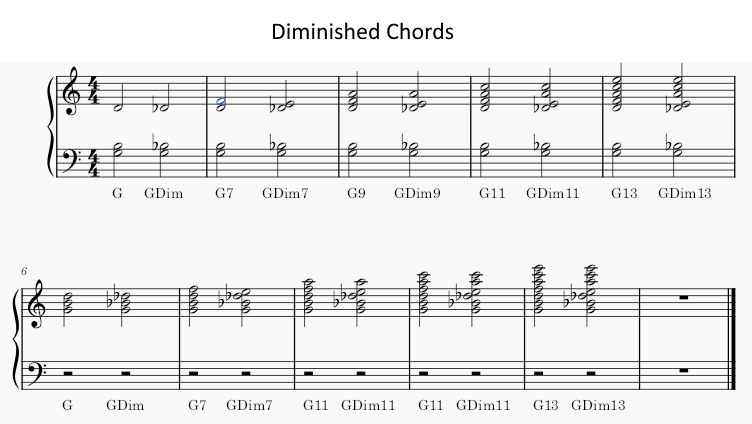
Diminished Chords
Can also be denoted as a small raised circle, °
Diminished chords have the third, fifth and seventh (if present) flattened. That means the already flattened 7th is flattened further.
The example of G13 here shows how the 13th is the same note as a diminished flat 7 (E). So a 13th chord cannot be truly changed into a diminished chord in the same way as the others, since the E is already present in the G13 chord. It is really a Gdim11, as it contains the same notes.
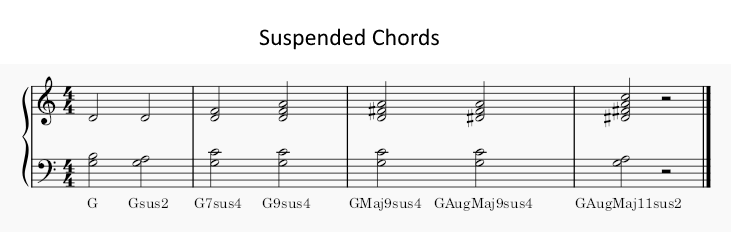
Suspended Chords
Suspended (“sus”) means that the third is to be removed. For example, “sus4” means remove the third and replace it with a 4th and “sus2” means remove the third and replace with a 2nd. Sometimes the third is removed but not replaced with anything and denoted by (no 3rd).
Common Alterations
Add the note if it isn’t already there and change the note if it is already there.
(♭ 9) – same note as ♭ 2
(#9) – same note as #2 or minor 3rd
(#11) – same note as #4
(♭ 6 )
(♭ 13) – same note as ♭ 6
(add 9)
(add “anything”)
Usually used when a note has been effectively skipped (often the 7th). Sometimes the word “add” is left out. For example, G(9) can be seen as G9 without the 7th.
6th Chords
Chords are named as “sixth chords” when they contain a 6th and not a 7th. They are not written with brackets or “add”. For example, “G6” or “Gm6”. When an additional note is added to the 6th chord (such as a 9th), it may be written as, for example, G6/9.
Notating a Chord with an Added Bass Note
This is done with a slash symbol then state the actual bass note (as oppose to the interval). For example. Gmaj7(#11) / F#
The “7alt” Term
This does not give you the exact chord. When you see “7alt”, you will need to listen to a recording of the piece to find out the chord. It refers to one of the base chords mentioned earlier (dominant chords) with an alteration. The “7” tells you that the chord contains a flat 7th. It will also contain a root note and a third.
Combinations Which Cannot Happen
Minor Augmented
The third in an augmented chord is a major third by definition of the augmented chord. A minor chord with an augmented fifth could be called as “Gm(#5)” but it is most likely to be spelled as a major chord. For the example of Gm, the raised fifth would create a D# major triad (in first inversion).
Minor Diminished
The third is already a minor third.
Minor Suspended
There is no 3rd in a suspended chord.
Inversions
The most important factor to remember about chord positions is that only the lowest note matters to determine the inversion. For example, the chord is said to be in root position if the root note is the lowest (the bass note). It does not mater where the others appear above it. If the third of the triad is the bass note, it is in first inversion. If the fifth is the bass note, it is a second inversion.
G63 = first inversion
G64 = second inversion
These confusing notations are still used sometimes. The 63 or 64 refers to the intervals resulting from the inversion. Fans of Figured Bass notation will enjoy using these, as the principle is the same. When looking at figure bass numbers under a score, you will see that the numbers correspond to intervals in relation to the bass note (with a few assumptions). An easier notation would be to simply use the backslash method described earlier and call the first inversion, “G / B” and the second inversion, “G / D”.
Just about any chord can be named in isolation using the rules discussed. However, many times a chord is given a particular name in context. Here are some examples:
More Confusion! “Augmented 6th Chords” – Italian, French, German
As mentioned earlier, augmented chords are those with the 5th augmented (raised). However, due to inconsistency in music notation, and augmented sixth chord is something different altogether. These three augmented sixth chords contain a root plus a note one augmented 6th above the root, as well as another note, one augmented sixth above that. Only, they tend to exist in a first inversion, where the root will be the middle note. The Italian contains only those three notes.
The French is the Italian chord with an added second (which is an augmented fourth from the lowest note).
The German is the Italian chord with an added minor third (which is a perfect fifth from the lowest note)
As you can see from the chords below, these augmented sixth chords could be given alternative names, according to the rules described earlier. In particular, the German chord is the same as a 7th chord (A♭ 7). The name given will depend on the context, the key, and how the chord resolves. Usually, the purpose of the augmented sixth chords is to resolve to the dominant (V) chord. In some cases it will resolve via a second inversion tonic (I) chord, especially the German because a progression to the dominant can result in parallel fifths (which is generally avoided).
Neapolitan Chord or Neapolitan Sixth
Sometimes denoted as “N6”. Once again, the “sixth” in the name of the Neapolitan is from the tradition of naming the first inversion by the 6th interval contained. The chord is actually made up of the notes of a normal major chord (in first inversion) but given the name “Neapolitan” because of the context.
Usually used in a minor key, the sub-dominant (IV) chord is altered whereby the fifth is raised by one semitone (the fifth is replaced)(#5). For example, in the key of Am, the Dm is altered from D, F, A to D, F, B♭. These are the notes of a B♭ major chord with the D as the bass, so the Neapolitan can also be called by ♭II63 (where the “63” means first inversion) or B♭/D.